Computational Contact Mechanics
This research concerns the development and analysis of finite element-based
methods for the solution of contact/impact problems between bodies that
may undergo large motions and deformations. Much of the work concentrates
on so-called ``dual'' formulations, that is those which employ Lagrange
multipliers to enforce the constraint(s) of impenetrability.
Emphasis is placed on the development of methodologies that are rigorous,
robust and suitable for large-scale computations.
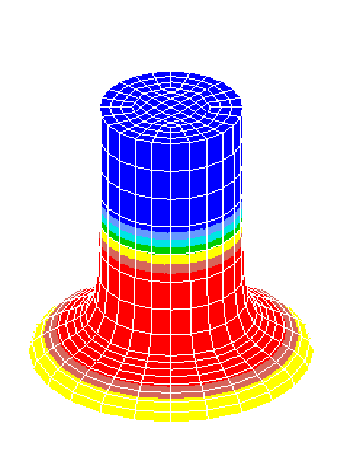
The following image is a plot of the true stress in the deformed
configuration resulting from a 3-D aluminum rod dynamically impacting
a rigid wall. The material is modeled as a finitely deforming rate-independent
elastic-plastic solid.
Related Publications:
J.M. Solberg, R.E. Jones and P. Papadopoulos.
``A Family of Simple Two-pass Dual Formulations for the Finite Element
Solution of Contact Problems'',
Comp. Meth. Appl. Mech. Engrg., 196, pp. 782-802, (2007).
R.E. Jones and P. Papadopoulos.
``Simulating Anisotropic Frictional Response using Smoothly Interpolated
Traction Fields'',
Comp. Meth. Appl. Mech. Engrg., 195, pp. 588-613, (2006).
J.M. Solberg and P. Papadopoulos.
``An Analysis of Dual Formulations for the Finite Element Solution of Two-body
Contact Problems'',
Comp. Meth. Appl. Mech. Engrg., 194, pp. 2734-2780, (2005).
R.E. Jones and P. Papadopoulos.
``A Novel Three-Dimensional Contact Finite Element Based on
Smooth Pressure Interpolations'',
Int. J. Num. Meth. Engrg., 51, pp. 791-811, (2001).

